Phi in Quantum Mechanics
27/02/12 15:07
Ever since the early days of quantum physics, atoms have been known to resonate inside a cube instead of a freestanding sphere. This became apparent in the early 20th century when it was discovered atoms with an even number of electrons are more stable than odd numbers and that the most stable have eight electrons orbiting symmetrically in the “L shell” to form the corners of a cube.
From the cubic model of the atom was born the idea that space must itself be quantized as a cubic lattice. Within this quantized lattice, light and other electromagnetic energy then propagates in waves, oscillating around the center of the cubes until some of it cools and becomes trapped inside to form atomic standing waves.
The predominant theory used today to describe this lattice is Quantum Chromodynamics. It defines points in space as “flavored” quark fields that are linked or “glued” together by gluon fields, forming a virtual field of cubes. [1] Each edge of the cube can then be defined as 1.26 fermions in length to produce a cubic volume of 2 fermions. This is then stacked in powers of 24n to represent all space, which is then congruent with the Lie group E8 when viewed through 27-D Jordan algebra (Fig. 1). [2]
As energy flows through the lattice, it oscillates between quark and gluon fields to form waves around the quarks, which aggregate into the particles of atomic nuclei. Tessellating the lattice as waves is helpful in showing how electromagnetic waves are able to travel around atoms while also forming harmonically spaced electron shells. But it reveals something even more important – the alignment of sinusoidal waves in the lattice create a slight gap in the lattice that permits light and other electromagnetic waves to propagate freely. This gap is, in fact, a manifestation of the Divine Proportion.
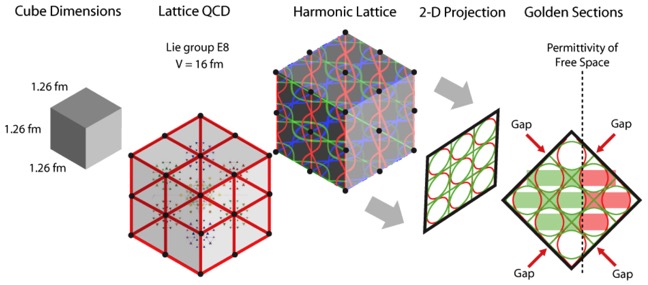
Figure 1. Permittivity of free space as a function of the golden ratio.
In quantum mechanics and quantum field theory, the ability of energy to travel freely through space is referred to as vacuum permittivity or the permittivity of free space and defined by the “electric constant.” Each point (or quark) in the lattice requires a little extra space in order to oscillate and resonate, which Phi provides in the phase-conjugate spacing of sinusoidal waves. Thus, the more harmonic and in-phase the vibration, the more the Phi gap comes into play and the more stable and coherent matter becomes. The study of these in-phase states is called quantum coherence and is the theoretical science behind such phenomena as lasers, superconductivity and superfluidity.
For instance, superfluidity results when helium-4 is cooled to the point it becomes highly coherent, producing something called Bose-Einstein condensate. In this exotic state of matter, atoms are said to resonate in-phase and within a common Schrödinger wave function. Here, the golden ratio plays a central role in encouraging coherence and enabling formation of highly stable atomic structures. In fact, a recent study found that coherent laser light could be used to cool calcium into a Bose-Einstein condensate with the greatest possible efficiency using a “golden ratio quasi-electrostatic 3-D lattice.” [3]
The golden ratio is also related to another mysterious constant of quantum coherence known as the fine-structure constant. As physicist Richard Feynman described it:
It has been a mystery ever since it was discovered over fifty years ago, and all good theoretical physicists put this number up on their wall and worry about it. Immediately you would like to know where this number for a coupling comes from: is it related to pi, or perhaps to the base of the natural logarithms? Nobody knows. It’s one of the greatest damn mysteries of physics: a magic number that comes to us with no understanding by man. You might say the “hand of God” wrote that number, and “we don’t know how he pushed his pencil.”
Since Feynman wrote this in 1985, it has since been shown that the fine-structure constant is probably just another instance of the golden ratio. As the “magic number” needed to calculate the electric constant and make space permeable, the fine-structure constant appears to be simply an expression of Phi-squared divided by 360. [4]
Fine-structure constant using Pi: e2 / ((h/2π) x c) = 1 / 137.036 = 0.00729
Fine-structure constant using Phi: Φ2/360 = 2.618/360 = 1 / 137.508 = 0.00727
In this study, researchers found that for any two atoms of the same kind, the covalent bond distance is the sum of its golden sections, which is then equal to the cationic radius of the atom.
It is the balance between Pi and Phi in the space lattice that enables all of this. Pi is the periodic constant of resonant energy while Phi is the stabilizing constant of damped space – together providing the coherence necessary for energy to propagate and atoms to resonate freely. Indeed, even the process of creating new waves can be expressed equally well using either Pi or Phi.
Heterodyning is how nature generates new waves from existing ones. Quantum mechanics typically models this by taking the mid-frequency between two Pi-periodic waves using the Schrödinger wave function. But in a 2008 paper by theoretical physicist Salvatore Giandinoto, it was shown to also be a result of recursive “Phi-heterodyning” and thus more fundamentally a property of space itself. [5] In this way, both energy and space can be said to be a manifestation of the Divine Proportion in the quantum structure of space. Perhaps this is the “hand of God” to which Dr. Feynman was referring.
Bibliography
1. Creutz, Michael (1985). Quarks, Gluons and Lattices. Cambridge University Press. ISBN 978-0521315357.
2. A. G. Lisi (2007). "An Exceptionally Simple Theory of Everything". arXiv:0711.0770
3. Adams C.S., Cox S.G., RIIS E., Arnold A.S., “Laser cooling of calcium in a ‘golden ratio’ quasi-electrostatic lattice,’ Journal of physics. B.Atomic, molecular and optical physics, Institute of Physics, Bristol, ISSN 0953-4075, CODEN HPAPEH, 2003, vol. 36, No 10, pp. 1933-1942.
4. Heyrovska, R., Narayan, S., et. al., “Fine-structure Constant, Anomalous Magnetic Moment, Relativity Factor and the Golden Ratio that Divides the Bohr Radius.” Doi:arXiv:physics/0509207; see http://arxiv.org/abs/physics/0509207.
5. Giandinoto, S., “Incorporation of the Golden Ratio Phi into the Schrödinger Wave Function using the Phi Recursive Heterodyning Set,” Advanced Laser Qauntum Dynamics Research Institute (ALQDRI), 10321 Briar Hollow Drive, St. Louis, MO 63146 USA.
From the cubic model of the atom was born the idea that space must itself be quantized as a cubic lattice. Within this quantized lattice, light and other electromagnetic energy then propagates in waves, oscillating around the center of the cubes until some of it cools and becomes trapped inside to form atomic standing waves.
The predominant theory used today to describe this lattice is Quantum Chromodynamics. It defines points in space as “flavored” quark fields that are linked or “glued” together by gluon fields, forming a virtual field of cubes. [1] Each edge of the cube can then be defined as 1.26 fermions in length to produce a cubic volume of 2 fermions. This is then stacked in powers of 24n to represent all space, which is then congruent with the Lie group E8 when viewed through 27-D Jordan algebra (Fig. 1). [2]
As energy flows through the lattice, it oscillates between quark and gluon fields to form waves around the quarks, which aggregate into the particles of atomic nuclei. Tessellating the lattice as waves is helpful in showing how electromagnetic waves are able to travel around atoms while also forming harmonically spaced electron shells. But it reveals something even more important – the alignment of sinusoidal waves in the lattice create a slight gap in the lattice that permits light and other electromagnetic waves to propagate freely. This gap is, in fact, a manifestation of the Divine Proportion.

Figure 1. Permittivity of free space as a function of the golden ratio.
In quantum mechanics and quantum field theory, the ability of energy to travel freely through space is referred to as vacuum permittivity or the permittivity of free space and defined by the “electric constant.” Each point (or quark) in the lattice requires a little extra space in order to oscillate and resonate, which Phi provides in the phase-conjugate spacing of sinusoidal waves. Thus, the more harmonic and in-phase the vibration, the more the Phi gap comes into play and the more stable and coherent matter becomes. The study of these in-phase states is called quantum coherence and is the theoretical science behind such phenomena as lasers, superconductivity and superfluidity.
For instance, superfluidity results when helium-4 is cooled to the point it becomes highly coherent, producing something called Bose-Einstein condensate. In this exotic state of matter, atoms are said to resonate in-phase and within a common Schrödinger wave function. Here, the golden ratio plays a central role in encouraging coherence and enabling formation of highly stable atomic structures. In fact, a recent study found that coherent laser light could be used to cool calcium into a Bose-Einstein condensate with the greatest possible efficiency using a “golden ratio quasi-electrostatic 3-D lattice.” [3]
The golden ratio is also related to another mysterious constant of quantum coherence known as the fine-structure constant. As physicist Richard Feynman described it:
It has been a mystery ever since it was discovered over fifty years ago, and all good theoretical physicists put this number up on their wall and worry about it. Immediately you would like to know where this number for a coupling comes from: is it related to pi, or perhaps to the base of the natural logarithms? Nobody knows. It’s one of the greatest damn mysteries of physics: a magic number that comes to us with no understanding by man. You might say the “hand of God” wrote that number, and “we don’t know how he pushed his pencil.”
Since Feynman wrote this in 1985, it has since been shown that the fine-structure constant is probably just another instance of the golden ratio. As the “magic number” needed to calculate the electric constant and make space permeable, the fine-structure constant appears to be simply an expression of Phi-squared divided by 360. [4]
Fine-structure constant using Pi: e2 / ((h/2π) x c) = 1 / 137.036 = 0.00729
Fine-structure constant using Phi: Φ2/360 = 2.618/360 = 1 / 137.508 = 0.00727
In this study, researchers found that for any two atoms of the same kind, the covalent bond distance is the sum of its golden sections, which is then equal to the cationic radius of the atom.
It is the balance between Pi and Phi in the space lattice that enables all of this. Pi is the periodic constant of resonant energy while Phi is the stabilizing constant of damped space – together providing the coherence necessary for energy to propagate and atoms to resonate freely. Indeed, even the process of creating new waves can be expressed equally well using either Pi or Phi.
Heterodyning is how nature generates new waves from existing ones. Quantum mechanics typically models this by taking the mid-frequency between two Pi-periodic waves using the Schrödinger wave function. But in a 2008 paper by theoretical physicist Salvatore Giandinoto, it was shown to also be a result of recursive “Phi-heterodyning” and thus more fundamentally a property of space itself. [5] In this way, both energy and space can be said to be a manifestation of the Divine Proportion in the quantum structure of space. Perhaps this is the “hand of God” to which Dr. Feynman was referring.
Bibliography
1. Creutz, Michael (1985). Quarks, Gluons and Lattices. Cambridge University Press. ISBN 978-0521315357.
2. A. G. Lisi (2007). "An Exceptionally Simple Theory of Everything". arXiv:0711.0770
3. Adams C.S., Cox S.G., RIIS E., Arnold A.S., “Laser cooling of calcium in a ‘golden ratio’ quasi-electrostatic lattice,’ Journal of physics. B.Atomic, molecular and optical physics, Institute of Physics, Bristol, ISSN 0953-4075, CODEN HPAPEH, 2003, vol. 36, No 10, pp. 1933-1942.
4. Heyrovska, R., Narayan, S., et. al., “Fine-structure Constant, Anomalous Magnetic Moment, Relativity Factor and the Golden Ratio that Divides the Bohr Radius.” Doi:arXiv:physics/0509207; see http://arxiv.org/abs/physics/0509207.
5. Giandinoto, S., “Incorporation of the Golden Ratio Phi into the Schrödinger Wave Function using the Phi Recursive Heterodyning Set,” Advanced Laser Qauntum Dynamics Research Institute (ALQDRI), 10321 Briar Hollow Drive, St. Louis, MO 63146 USA.


